Next: 4.3 Magnetization reversal in Up: 4. Magnetization reversal in Previous: 4.1 Experimental motivation Contents
|
We have modeled the field induced reversal
processes taking place in Fe particles having dimensions in the
range ![]() (thickness)
(thickness)
![]() (width)
(width)
![]() (length). The particle dimensions range, although not covering completely the experimentally observed particle size
scale, is affordable from the point of view of the computing time. Due to the above mentioned
isolated character of the Fe ribbons, we assume that the behavior of
the samples can be well represented by that of an isolated simulated
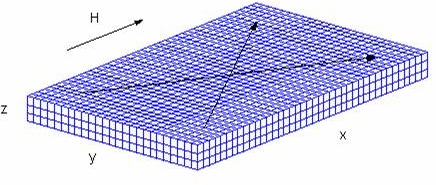
ribbon. We considered the occurrence in the particles of a (110) texture and, therefore, easy axes oriented along directions forming 45
(length). The particle dimensions range, although not covering completely the experimentally observed particle size
scale, is affordable from the point of view of the computing time. Due to the above mentioned
isolated character of the Fe ribbons, we assume that the behavior of
the samples can be well represented by that of an isolated simulated
ribbon. We considered the occurrence in the particles of a (110) texture and, therefore, easy axes oriented along directions forming 45![]() with the applied field direction and the ribbon long axis (see Fig. 4.4). The particles were discretized in elements of size
with the applied field direction and the ribbon long axis (see Fig. 4.4). The particles were discretized in elements of size ![]() (
(![]() of the exchange correlation length of Fe,
of the exchange correlation length of Fe,
![]() ).
A particular relaxation stage was terminated
when achieved a value lower than
).
A particular relaxation stage was terminated
when achieved a value lower than ![]() for the error (see Section 2.2.3). The hysteresis cycles were
followed by starting with the application of a
for the error (see Section 2.2.3). The hysteresis cycles were
followed by starting with the application of a ![]() field which was
always enough to achieve the saturation. The field was varied
through variable size increments which were large in the saturation
region and of the order of
field which was
always enough to achieve the saturation. The field was varied
through variable size increments which were large in the saturation
region and of the order of
![]() in the coercive force region.
The considered Fe parameters were
biaxial anisotropy value
in the coercive force region.
The considered Fe parameters were
biaxial anisotropy value
![]() , micromagnetic exchange value
, micromagnetic exchange value
![]() and saturation magnetization value
and saturation magnetization value
![]() and were taken from [Skomski 99, Page 158].
and were taken from [Skomski 99, Page 158].
2008-04-04