Next: 1 Evaluation of the Up: 3 "Ab-initio" modeling of Previous: 4 Antisymmetric anisotropic exchange Contents
The magneto-crystalline anisotropy (MCA) energy has its origin in the spin-orbit coupling under the ordering imposed by the crystalline lattice of the material.
In the most general case, the magnetocrystalline anisotropy matrix ![]() can be written as a function of its eigenvectors (corresponding to the easy axes)
can be written as a function of its eigenvectors (corresponding to the easy axes)
![]() and eigenvalues
and eigenvalues
![]() :
:
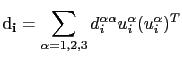 |
(109) |
Epitaxially grown systems present usually uniaxial anisotropy. If we suppose that in this case the easy axis is parallel to the Z axis, then the on-site anisotropy has the form:
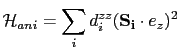 |
(111) |
We have only described the on-site magneto-crystalline anisotropy. However, there could exist other contributions to the magnetic anisotropy of the system, as e.g. the two-site magnetic anisotropy, mentioned already above and discussed later in this chapter.